Adding Integers Using Algebra Tiles -
Middle School / Math / Algebra
A lesson from Michael Waski of The Math Institute in adding integers using algebra tiles. Head over to The Math Institute website today to see how we can help enhance your classroom experience! https://www.greatworkinc.org/adolescent-math-institute-1
Hi! Today?s video is about adding integers. Oftentimes, when we think about adding integers student are told to memorize these two rules. When the signs are the same, add, and keep the sign. When the signs are different, subtract, and keep the sign of the larger number. The problem with these rules is that if students don?t use them for a while, they could easily forget them. And if they do happen to remember them, they often get them mixed up when they have new rules for things like subtraction, and multiplication, and division.
So, with working with the algebra tiles, students can have a deeper understanding of what addition actually is. And they won?t forget the rule because really, they don?t have to memorize anything. They really understand what they?re doing and they are able to add very easily.
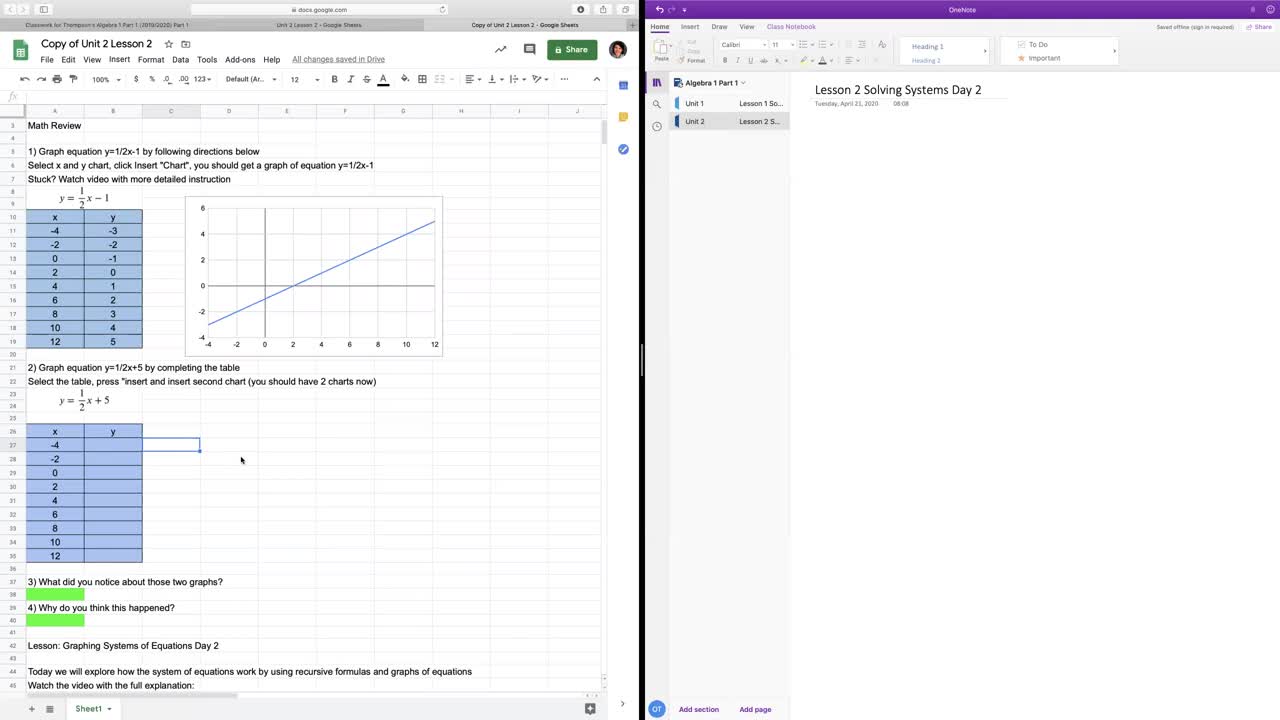
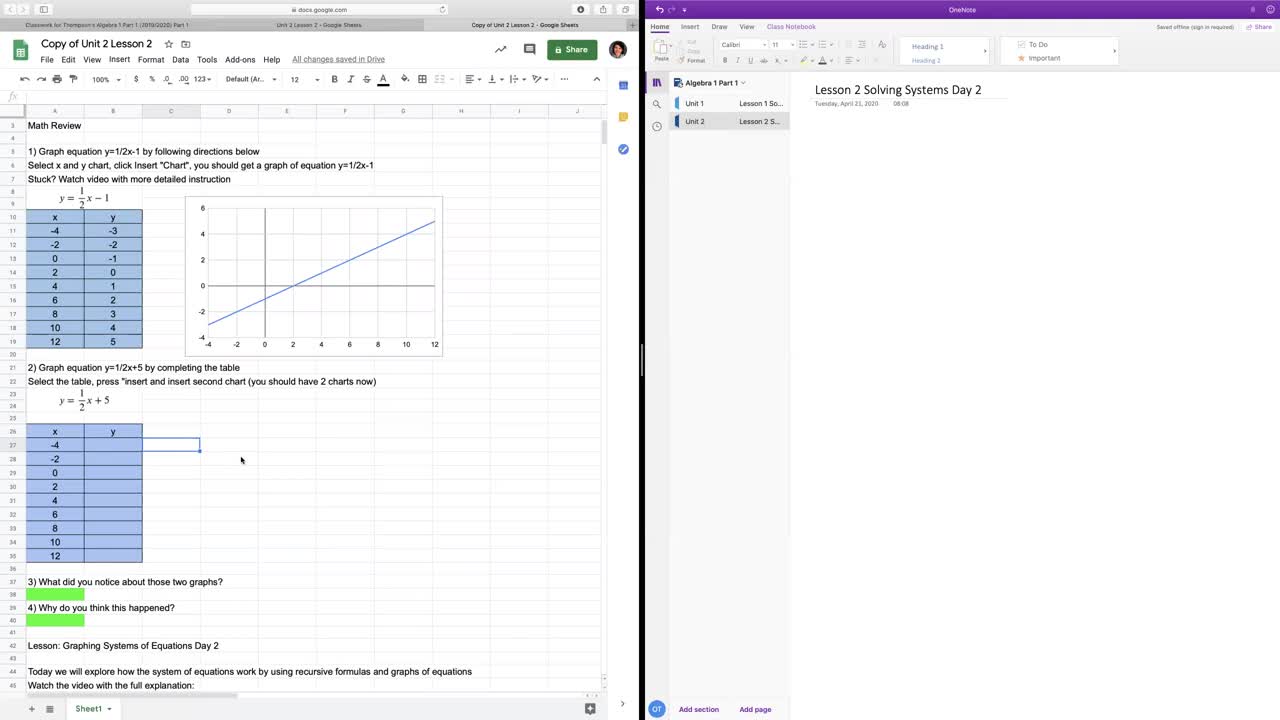
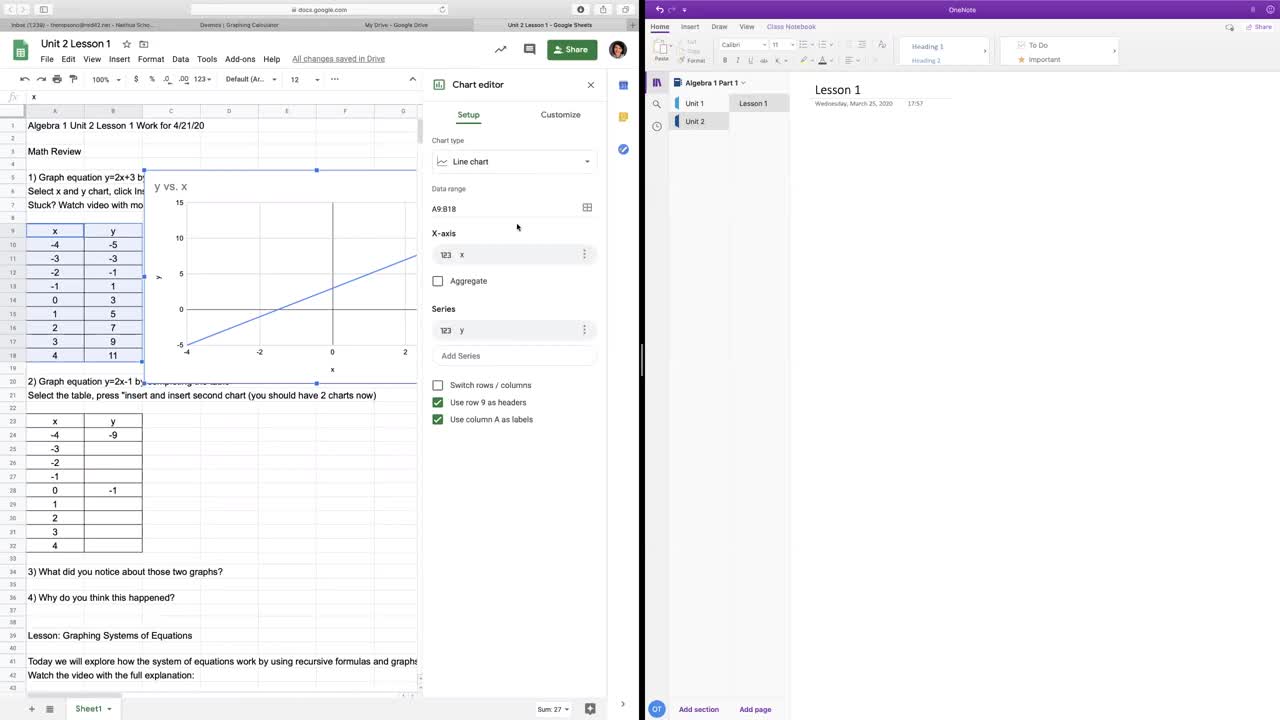
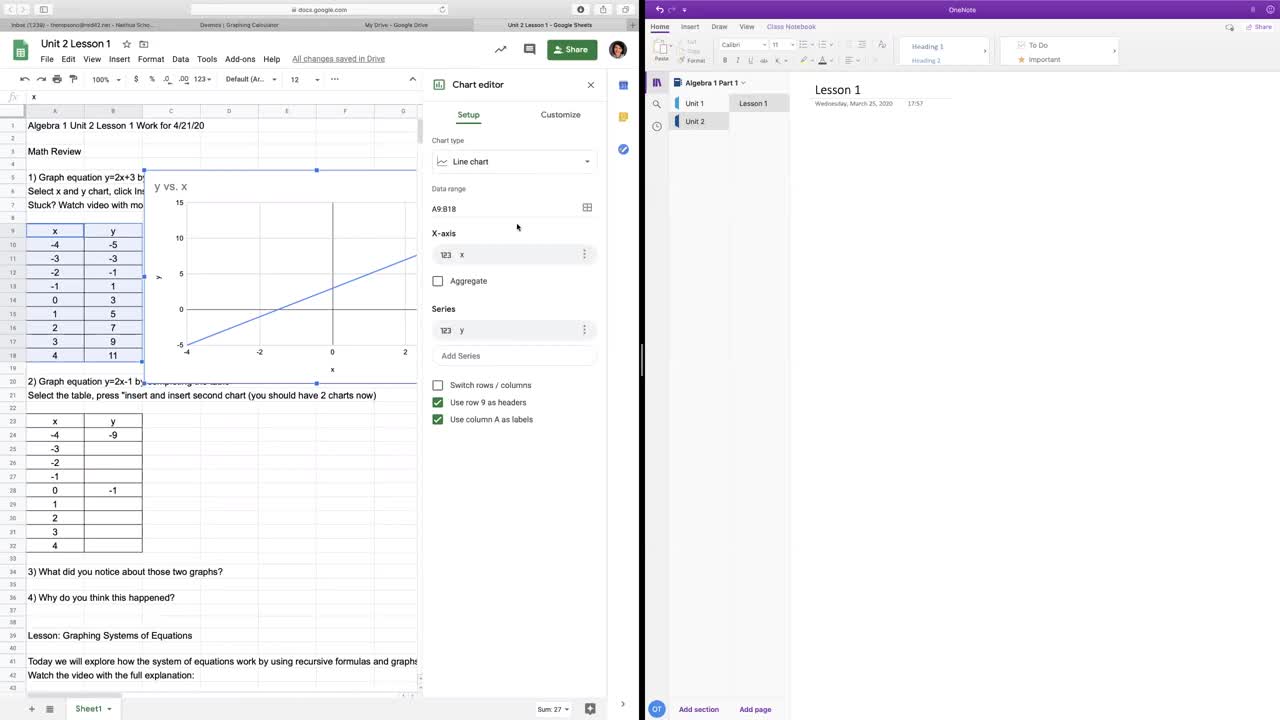
Let?s do a few examples. So, we can start with something simple like positive five plus positive three. Now we know the students know the answer to this and they should. But what we can do is remind them what is addition. Addition is putting things together. So, if you have a pile of money. And another person had another pile of money. And you wanted to know what we had altogether we just put them on a big pile, and we count them all up. So, that?s what we?re doing here.
Now we know the answer should be positive eight because we?ve been doing addition forever, but let?s see what it looks like using the algebra tiles. So, here?s our positive five over here. Here?s our positive three here. And we put them together and we just read the number that we have in front of us. And that indeed is positive eight.
Now, let?s do another example. Here we?ll start off with negative four plus negative two. And so, this negative four, we?ll represent this way. And then over here we?ll have negative two. And because it?s addition, what do we do? We combine these together and now we see what we have. And we just have negative six. So, negative four plus negative two is negative six. And again, all we had to do was just read the number in front of us. Just like we did in the original integer practice.
Now, let?s do one where we added integers with different signs. Here we have positive seven plus negative three. We?ll start off with a group of seven positives here. And three negatives here. Now once again, we just combine these together. And if students have done good work in the integer introduction, reading numbers with zero pairs, they will already know how to do this. They?ll make the zero pairs, take them away, and see what they have left. And this number is positive four. In a way, they?re not even adding. They?re just seeing what they have.
Let?s do one more example. Let?s try this last one. Negative six plus positive four. We?ll start off with six negatives and four positives. Combine them together because its addition. We?ll make our zero pairs, take those away, and see that our final answer is negative two.
So, with a problem like positive seven plus negative three, we want students to notice that there?s a cancellation happening here. And that whatever is in the negative pile is gonna cancel with some of the things in the positive pile. Or more generally, whatever?s in the smaller pile is gonna cancel with some of the things in the larger pile, and there?s always gonna be some of that larger pile left over. So, if they come to an example like positive twenty-eight plus negative four, they won?t have to actually do a rule or actually have the materials with them, they?ll just see that four of those negatives, is gonna cancel with four of those positives, and that means I?ll have twenty-four positives left over. And it?s pretty simple when you look at it that way. And that?s what students will eventually see.
So, besides having a great mental model through addition, as students continue to work, they?re gonna make some observations. When students usually do addition, they end up with more of whatever they started with. But sometime here they end up with less. Now, usually the only way that you could end up with less of something is through subtraction, but here, they end up with less when they start adding the opposite. And students are making this connection between having less of something and adding the opposite. Which is how we?re gonna introduce the idea of subtraction later on. And so, working with these materials not only help students with addition, but really gives them a leg up on subtraction. And that?s gonna be part of another video.
So, thanks for watching.
So, with working with the algebra tiles, students can have a deeper understanding of what addition actually is. And they won?t forget the rule because really, they don?t have to memorize anything. They really understand what they?re doing and they are able to add very easily.
Let?s do a few examples. So, we can start with something simple like positive five plus positive three. Now we know the students know the answer to this and they should. But what we can do is remind them what is addition. Addition is putting things together. So, if you have a pile of money. And another person had another pile of money. And you wanted to know what we had altogether we just put them on a big pile, and we count them all up. So, that?s what we?re doing here.
Now we know the answer should be positive eight because we?ve been doing addition forever, but let?s see what it looks like using the algebra tiles. So, here?s our positive five over here. Here?s our positive three here. And we put them together and we just read the number that we have in front of us. And that indeed is positive eight.
Now, let?s do another example. Here we?ll start off with negative four plus negative two. And so, this negative four, we?ll represent this way. And then over here we?ll have negative two. And because it?s addition, what do we do? We combine these together and now we see what we have. And we just have negative six. So, negative four plus negative two is negative six. And again, all we had to do was just read the number in front of us. Just like we did in the original integer practice.
Now, let?s do one where we added integers with different signs. Here we have positive seven plus negative three. We?ll start off with a group of seven positives here. And three negatives here. Now once again, we just combine these together. And if students have done good work in the integer introduction, reading numbers with zero pairs, they will already know how to do this. They?ll make the zero pairs, take them away, and see what they have left. And this number is positive four. In a way, they?re not even adding. They?re just seeing what they have.
Let?s do one more example. Let?s try this last one. Negative six plus positive four. We?ll start off with six negatives and four positives. Combine them together because its addition. We?ll make our zero pairs, take those away, and see that our final answer is negative two.
So, with a problem like positive seven plus negative three, we want students to notice that there?s a cancellation happening here. And that whatever is in the negative pile is gonna cancel with some of the things in the positive pile. Or more generally, whatever?s in the smaller pile is gonna cancel with some of the things in the larger pile, and there?s always gonna be some of that larger pile left over. So, if they come to an example like positive twenty-eight plus negative four, they won?t have to actually do a rule or actually have the materials with them, they?ll just see that four of those negatives, is gonna cancel with four of those positives, and that means I?ll have twenty-four positives left over. And it?s pretty simple when you look at it that way. And that?s what students will eventually see.
So, besides having a great mental model through addition, as students continue to work, they?re gonna make some observations. When students usually do addition, they end up with more of whatever they started with. But sometime here they end up with less. Now, usually the only way that you could end up with less of something is through subtraction, but here, they end up with less when they start adding the opposite. And students are making this connection between having less of something and adding the opposite. Which is how we?re gonna introduce the idea of subtraction later on. And so, working with these materials not only help students with addition, but really gives them a leg up on subtraction. And that?s gonna be part of another video.
So, thanks for watching.